
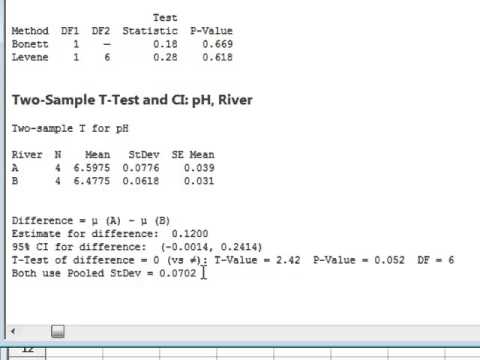
We can easily test the normality of the samples by creating a normal probability plot, however, verifying homogeneous variances can be more difficult. The assumptions for ANOVA are (1) observations in each treatment group represents a random sample from that population (2) each of the populations is normally distributed (3) population variances for each treatment group are homogeneous (i.e., ). Analysis of variance allows us to test the null hypothesis (all means are equal) against the alternative hypothesis (at least one mean is different) with a specified value of α. As the number of populations increases, the probability of making a Type I error using multiple t-tests also increases. A 14% probability of a Type I error is much higher than the desired alpha of 5% (remember: α is the same as Type I error). There is a 1 – 0.95 3 = 0.14 (14%) probability that at least one test will lead to an incorrect rejection of the null hypothesis. The probability that all three tests correctly do not reject the null hypothesis is 0.95 3 = 0.86. Each test would have a 95% probability of correctly not rejecting the null hypothesis.

If we used a 5% level of significance, each test would have a probability of a Type I error (rejecting the null hypothesis when it is true) of α = 0.05. If we continue this way, we would need to test three different pairs of hypotheses: H 0: µ 1= µ 2 It would be tempting to test this null hypothesis H 0: µ 1= µ 2= µ 3 by comparing the population means two at a time. H 1: at least one of the means is significantly different from the others The null and alternative hypotheses are: H 0: µ 1= µ 2= µ 3 The biologist would want to estimate the mean annual seed production under the three different treatments, while also testing to see which treatment results in the lowest annual seed production. The objects of ANOVA are (1) estimate treatment means, and the differences of treatment means (2) test hypotheses for statistical significance of comparisons of treatment means, where “treatment” or “factor” is the characteristic that distinguishes the populations.įor example, a biologist might compare the effect that three different herbicides may have on seed production of an invasive species in a forest environment. This characteristic is sometimes referred to as a treatment or factor.Ī treatment (or factor) is a property, or characteristic, that allows us to distinguish the different populations from one another.
This method is also referred to as single-factor ANOVA because we use a single property, or characteristic, for categorizing the populations. Analysis of variance (ANOVA) is an inferential method used to test the equality of three or more population means. This chapter examines methods for comparing more than two means. Previously, we have tested hypotheses about two population means.


 0 kommentar(er)
0 kommentar(er)
